Fill in the blanks

The result tan^–1 x – tan^–1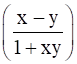 is true when value of xy is _________.

The result tan^–1 x – tan^–1 is true when value of xy is > -1.

We have,

Principal range of tan^-1a is

Let tan^-1x = A and tan^-1y = B … (1)

So, A,B ϵ

We know that, … (2)

From (1) and (2), we get,

Applying, tan^-1 both sides, we get,

As, principal range of tan^-1a is .

So, for tan^-1tan(A-B) to be equal to A-B,

A-B must lie in – (3)

Now, if both A,B < 0, then A, B ϵ

∴ A ϵ and -B ϵ

So, A – B ϵ

So, from (3),

tan^-1tan(A-B) = A-B

Now, if both A,B > 0, then A, B ϵ

∴ A ϵ and -B ϵ

So, A – B ϵ

So, from (3),

tan^-1tan(A-B) = A-B

Now, if A > 0 and B < 0,

Then, A ϵ and B ϵ

∴ A ϵ and -B ϵ

So, A – B ϵ (0,π)

But, required condition is A – B ϵ

As, here A – B ϵ (0,π), so we must have A – B ϵ

Applying tan on both sides,

As,

So, tan A < - cot B

Again,

So,

⇒ tan A tan B < -1

As, tan B < 0

xy > -1

Now, if A < 0 and B > 0,

Then, A ϵ and B ϵ

∴ A ϵ and -B ϵ

So, A – B ϵ (-π,0)

But, required condition is A – B ϵ

As, here A – B ϵ (0,π), so we must have A – B ϵ

Applying tan on both sides,

As,

So, tan B > - cot A

Again,

So,

⇒ tan A tan B > -1

⇒xy > -1