Using the properties of determinants in prove that:

Question

Philoid · Accepted Answer

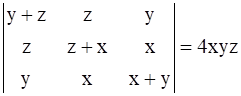
Taking LHS,

By applying R₁→ R₁ + R₂ + R₃, we get

Taking 2 common from the first row, we get

Applying R₁→ R₁ – R₂, we get

Applying R₃→ R₃ - R₁, we get

Applying R₂→ R₂ – R₁, we get

Taking y, z, x common from R₁, R₂ and R₃ respectively, we get

Expanding along C₁, we get

= 2xyz [(1){(1) – 0} – (1){0 – 1} + 0}]

= 2xyz [1 + 1]

= 4xyz

= RHS

Hence,

∴ LHS = RHS

Hence Proved