If A, B and C are angles of a triangle, then the determinant 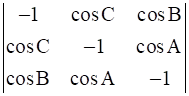is equal to

We have,

Expanding along C₁, we get

= [(-1){1 – cos²A} – cos C{-cos C – cos Acos B} + cos B{cos A cos C + cos B}]

= -1 + cos²A + cos²C + cos A cos B cos C + cos A cos B cos C + cos²B

= -1 + cos²A + cos²B + cos²C + 2cos A cos B cos C

Here, we use formula

1 + cos2A = 2cos²A

Taking L.C.M, we get

Now, we use the formula:

cos(A + B) cos(A – B) = 2cos Acos B

so, cos2A + cos2B = 2 cos(A + B) cos(A – B)

…(i)

Since, A, B and C are the angles of a triangle and we know that the sum of the angles of a triangle = 180° or π

⇒ A + B + C = π

⇒ A + B = π – C

Putting the value of (A+B) in eq. (i), we get

[∵ cos(π – x) = -cos X]

= -cos C{cos(A – B) – cos C} + 2cos Acos Bcos C

= -cos C[cos(A – B) – cos{π – (A + B)}] + 2cos Acos Bcos C

= -cos C[cos(A – B) + cos(A + B)] + + 2cos Acos Bcos C

= -cos C[2cos Acos B] + 2cos Acos Bcos C

= 0