If x, y, z are all different from zero and 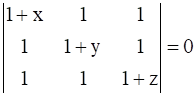, then value of x -1 + y -1 + z -1 is

Question

Philoid · Accepted Answer

Given

Applying C₁→ C �₁ – C₃ and C₂→ C₂ – C₃,

⇒

Expanding along R₁,

⇒ x [y (1 + z) + z] – 0 + 1 (yz) = 0

⇒ xy + xyz + xz + yz = 0

Dividing by xyz on both sides,

Hence, option (D) satisfies.

If x, y, z are all different from zero and , then value of x-1 + y-1 + z-1 is