Prove that has maximum value at

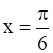
Given: f(x) = sin X + √3 cos X

To prove: the given function has maximum value at

Explanation: given f(x) = sin X + √3 cos X

We will find the first derivative of the given function, we get

Now applying the derivative, we get

f'(x) = cos x-√3sin x

Now critical point is found by equating the first derivative to 0, i.e.,

f’(x) = 0

⇒ cos x-√3sin x = 0

⇒ √3sin x = cos x

This is possible only when

Now the second derivative of the function is

Applying the derivative, we get

f’’(x) = -sin x-√3cos x

Now we will substitute in the above equation, we get

f’’(x) = -sin x-√3cos x

Substituting the corresponding value, we get

Hence f(x) has maximum value at .

Hence proved