The maximum value of 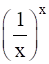is:

Let ………(i)

Taking logarithm on both sides we get

Now applying first derivative, we get

Now applying the product rule of differentiation we get

Now applying the derivative we get

Substituting the value of y from equation (i), we get

Now we will find the critical point by equating equation (i) to 0, we get

as cannot be equal to 0

But 1=log e¹

Equating the terms we get

Hence f(x) has a stationary point at .

i.e the maximum value of

So the correct option is option C.