Fill in the blanks in each of the following

The function 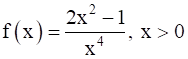decreases in the interval _______.

Given

Now apply the derivative we get

Now applying the quotient rule of differentiation and the differentiation of the constant term is 0 we get

We will equate this with 0 to get critical points,

f’(x)=0

⇒ x²-1=0

⇒ x²=1

⇒ x=±1

The intervals formed by these two critical numbers are (-∞, -1), (-1, 0), (0, 1) and (1, ∞)

(i) in the interval (-∞, -1), f’(x)>0

∴ f(x) is increasing in (-∞,-1)

(ii) in the interval (-1, 0), f’(x)<0

∴ f(x) is decreasing in(-1,0)

(iii) in the interval (0, 1), f’(x)>0

∴ f(x) is increasing in (1, ∞)

(iii) in the interval (1, ∞), f’(x)<0

∴ f(x) is decreasing in (1, ∞)

Hence the function decreases in the interval (1, ∞).