Fill in the blanks in each of the following

The least value of the function 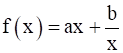(a > 0, b > 0, x > 0) is ______.

Given (a > 0, b > 0, x > 0)

Now apply the derivative we get

Now applying the sum rule of differentiation we get

Now applying the quotient rule of differentiation on second part we get

We will equate this with 0 to get critical points,

f’(x)=0

Now second derivative gives,

Now apply the derivative we get

Now applying the sum rule of differentiation we get

Now applying the quotient rule of differentiation on second part we get

We will equate this with we get

Thus the least value of f(x) is

Multiply and divide by , we get

Hence the least value of the function (a > 0, b > 0, x > 0) is