Prove: 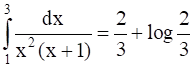
Given: ![]()

![]()
Using partial differentiation:
![]()

![]()
⇒ 1 = Ax2 +Ax+ B+Bx+ Cx2
⇒ 1 = B + (A+B)x + (A+C)x2
Equating the coefficients of x, x2 and constant value. We get:
(a) B = 1
(b) A + B = 0 ⇒ A = -B ⇒ A = -1
( c) A + C =0 ⇒ C = -A ⇒ C = 1
Put these values in equation (1)
![]()
![]()
![]()


![]()
![]()
![]()
![]()
⇒ L.H.S = R.H.S
Hence proved.
34