Find the equation of the line which is perpendicular to the line 3x + 2y = 8 and passes through the midpoint of the line joining the points (6, 4) and (4, - 2).

Question

Philoid · Accepted Answer

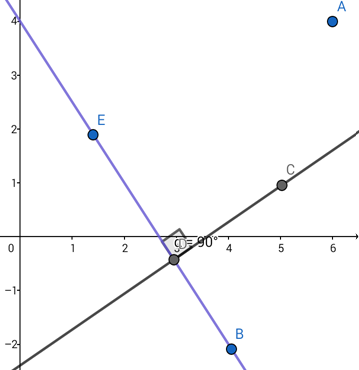
Given: The given line is 3x + 2y = 8. The perpendicular line passes through the midpoint of (6,4) and (4, - 2).

Formulae to be used: The product of slopes of two perpendicular lines = - 1.

If (a,b) and (c,d) be two points, then their midpoint is given by

The slope of this line is .

the slope of the perpendicular line =

The equation of the line can be written in the form

(c is the y - intercept)

This line passes through the midpoint of (6,4) and (4, - 2).

The co - ordinates of the midpoint of the line joining the given points is

(5,1) satisfies the equation

The required equation is

i.e. 2x - 3y = 7