If 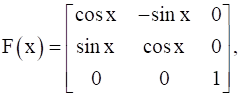show that F(x) . F(y) = F(x + y).

Given :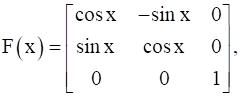

To show : F(x) . F(y) = F(x + y).

Formula used :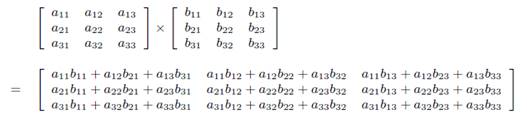

If A is a matrix of order a b and B is a matrix of order c d ,then matrix AB exists and is of order a d ,if and only if b = c

F(x) =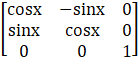

F(y) =

F(x + y) =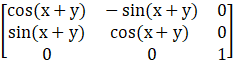

F(x) . F(y) = .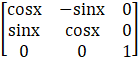

=

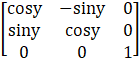
F(x) . F(y) =

We know that,

cosx(cosy) – sinx (siny) = cos(x+y) and -cosx(siny) - sinx(cosy) = -sin(x+y)

F(x) . F(y) =

F(x + y) = F(x) . F(y) =

F(x + y) = F(x) . F(y)