Mark the correct alternative in the following:

A vector parallel to the line of intersection of planes and is

The two planes are, and

The line of intersection of planes and

is parallel to

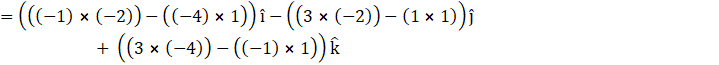
The line of intersection of planes and

is parallel to

Alternative:

The two planes are, and

or, 3x - y + z=1 and x - 4y - 2z=2

Putting, z=k, we get,

3x - y + k=1 ………………………. (1)

and x - 4y - 2k=2 …………………… (2)

Multiplying equation (2) by 3 and then subtracting equation (1) from it, we get,

3(x - 4y - 2k) - (3x - y + k)=(3×2) - 1

3x - 12y - 6k - 3x + y - k=6 - 1

- 11y - 7k=5

Substituting y, in equation (1) we get,

33x + 5 + 7k + 11k=11

18k=11 - 5 - 33x

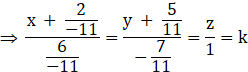
Equation of the line of intersection,

The line of intersection of planes and

is parallel to .