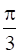Given the unit vector makes,
• an angle of ![]() with x-axis
with x-axis
• an angle of ![]() with y-axis
with y-axis
• an angle of θ with z-axis
• θ is acute angle
Let the unit vector ![]() be:
be: ![]()
As given it is a unit vector,
Therefore ![]() = 1
= 1
As the angle between in ![]() and x-axis is
and x-axis is ![]() , the scalar product of the vectors can be performed.
, the scalar product of the vectors can be performed.
The scalar product of the two vectors is given by
![]()
![]()
![]()
[as both the vectors are of magnitude 1].
![]()
![]()
![]()
As the angle between in ![]() and y-axis is
and y-axis is ![]() , the scalar product of the vectors can be performed.
, the scalar product of the vectors can be performed.
![]()
![]()
![]()
![]()
![]()
Similarly the angle between in ![]() and y-axis is θ , the scalar product of the vectors can be performed.
and y-axis is θ , the scalar product of the vectors can be performed.
![]()
![]()
![]()
![]()
![]()
The magnitude of a vector x![]() + y
+ y![]() + z
+ z ![]() is given by
is given by ![]() .
.
Now consider the magnitude of the vector ![]()
1 ![]()
1 ![]()
[Squaring on both sides]
1 = ![]()
cos2θ ![]()
cos2θ = ![]()
cos θ = ![]()
cosθ = ![]()
As given in the question θ is acute angle, so θ belongs to 1st quadrant and is positive.
Therefore![]()