Find 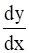 , when
, when
Theorem: y and x are given in a different variable that is θ . We can find ![]() by finding
by finding ![]() and
and ![]() and then dividing them to get the required thing.
and then dividing them to get the required thing.
![]() =
= ![]()
= a× 3 sin2θ × cosθ (using the chain rule ![]() = 3sin2θ×
= 3sin2θ× ![]() = 2sin2θ × cosθ )
= 2sin2θ × cosθ )
= 3asin2θcosθ . ………..(1)
![]()
= a × (3cos2θ)× (-sinθ ) (using chain rule ![]() = 2cosθ×
= 2cosθ× ![]() = 2 cosθ × (-sinθ ) )
= 2 cosθ × (-sinθ ) )
= -3asinθcos2θ.
Dividing (1) and (2), we get
![]() =
= ![]()
= ![]() .
.
= -tanθ
1