Show that the four points with position vectors ![]() and
and ![]() are coplanar.
are coplanar.
Given :
Let A, B, C & D be four points with position vectors ![]() .
.
Therefore,
![]()
![]()
![]()
![]()
To Prove : Points A, B, C & D are coplanar.
Formulae :
1) Vectors :
If A & B are two points with position vectors ![]() ,
,
Where,
![]()
![]()
then vector ![]() is given by,
is given by,
![]()
![]()
2) Scalar Triple Product:
If
![]()
![]()
![]()
Then,
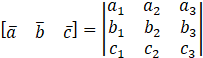
3) Determinant :
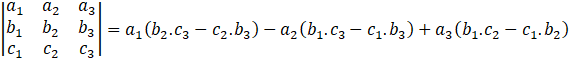
Answer :
For given position vectors,
![]()
![]()
![]()
![]()
Vectors ![]() are given by,
are given by,
![]()
![]()
![]() ………eq(1)
………eq(1)
![]()
![]()
![]() ………eq(2)
………eq(2)
![]()
![]()
![]() ………eq(3)
………eq(3)
Now, for vectors
![]()
![]()
![]()
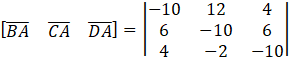
![]()
= -10(112) – 12(-84) + 4(28)
= -1120 + 1008 + 112
= 0
![]()
Hence, vectors ![]() are coplanar.
are coplanar.
Therefore, points A, B, C & D are coplanar.
Note : Four points A, B, C & D are coplanar if and only if ![]()
1