Find the vector and Cartesian equations of a plane containing the two lines 
and 
Also show that the lines 
lies in the plane.
Given : Equations of lines -
To Prove : 
are coplanar.
To Find : Equation of plane.
Formulae :
1) Cross Product :
If 
are two vectors
then,
2) Dot Product :
If 
are two vectors
then,
3) Coplanarity of two lines :
If two lines 
are coplanar then
4) Equation of plane :
If two lines 
are coplanar then equation of the plane containing them is
Where,
Answer :
Given equations of lines are
Let, 
Where,
Now,
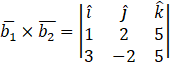
Therefore,
= 40 + 10 + 24
= 74
……… eq(1)
And
= 60 + 30 – 16
= 74
……… eq(2)
From eq(1) and eq(2)
Hence lines 
are coplanar.
Equation of plane containing lines 
is
Now,
From eq(1)
4
Therefore, equation of required plane is
This vector equation of plane.
As 
= 20x + 10y – 8z
Therefore, equation of plane is
20x + 10y – 8z = 74
20x + 10y – 8z – 74 = 0
10x + 5y – 4z – 37 = 0
This Cartesian equation of plane.