Air is pumped into the tubes of a cycle rickshaw at a pressure of 2 atm. The volume of each tube at this pressure is 0.002 m³. One of the tubes gets punctured and the volume of the tube reduces to 0.0005 m³. How many moles of air have leaked out? Assume that the temperature remains constant at 300K and that the air behaves as an ideal gas.

Question

Philoid · Accepted Answer

We know ideal gas equation

PV=nRT

Where V= volume of gas

R=gas constant=8.31Jmol^-1K^-1

T=temperature

n=number of moles of gas

P=pressure of gas

Given

Pressure inside the tyre P₁=2atm=2Pa

Volume at P₁, V₁=0.002m³

Reduced volume V₂=0.0005m³

Temperature remains constant so T₁=T₂=300K

Let when the gas is leaked out the pressure P₂ becomes equal to atmospheric pressure. So P₂=1.0Pa.

Number of moles initially n₁

Similarly

Final number of moles n₂

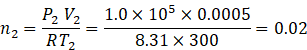
So, number of moles leaked out will be n₁-n₂=0.16-0.02=0.14.