A composite slab is prepared by pasting two plates of thicknesses L₁ and L₂ and thermal conductivities K₁ and K₂. The slabs have equal cross-sectional area. Find the equivalent conductivity of the composite slab.

Here the slabs are placed in such a way that their conductivities are in series as shown in the fig below

Given

Thicknesses of the slabs as L₁ and L₂

Thermal conductivities as K₁ and K₂.

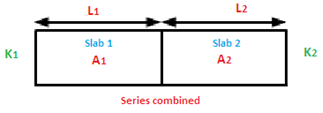
Hence, their equivalent conductivity is similar to 2 resistors connected in series.

From above fig.

Given thermal conductivities of the slabs as K₁ andK₂

The of rate thermal conduction through first slab is given by -

Q_{1 =} (1)

Where

= thermal conductivity of the first slab

= area of first slab

= length of first slab

= temperature of the first slab and

T= junction temperature

Similarly the rate thermal conduction through second slab is given by –

Q₂ = (2)

Since they are connected end to end

Q_eqv = Q₁ = Q₂

Where Q_eqv is given by

Q_eqv =

Also, their area of cross section are equal ie,

A₁ = A₂ (3)

From (1) ,(2) and (3)

==

Solving for T

T = 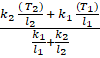(4)

Substituting (4) in (1) and (2) –

K_{eqv =}