Using B = μ₀ H find the ratio E₀/H₀ for a plane electromagnetic wave propagating through vacuum. Show that is has the dimensions of electric resistance. This ratio is a universal constant called the impedance of free space.

Given: The magnetic field density and the magnetic field strength

as related by the relation

where B is the magnetic field density, μ_m is the relative magnetic

permeability of the material and depends on the nature of the

material or the medium and H is the magnetic field strength which

tells upto what extent a material can be magnetized.

For free space, the relation becomes

…. (i)

where B₀ is the field intensity in free space(vacuum), H₀ is the

strength in free space and μ₀ is the magnetic permeability of free

space. μ₀ is a universal constant and its value is 4π × 10^-7 T m A^-1.

We can define a relation between the electric field and magnetic field

of an electromagnetic wave travelling in vacuum. It is

…. (ii)

where E₀ is the electric field density and C is the speed of light in

vacuum.

From (i) and (ii)

…. (iii)

To find the dimension of , we will find the dimension of the

quantity in the R.H.S.

The dimension of C is [L T^-1] because it is the speed.

To find the dimension of , we need to consider the Biot Savart’s

law which gives the magnetic field due to a current carrying

conductor. According to Biot Savart law

…. (iv)

where i is the current in the conductor, L is the length of conductor and R is the distance between conductor and point where field is to be found.

The scalar form of the above equation will be simply

…. (v)

From (v)

the dimensions of μ₀ will be the dimensions of R.H.S

dimension of B= [M T^-2 A �^-1]

dimension of R²=[L²]

dimension of L=[L]

dimension of i=[A]

dimension of R.H.S

Therefore dimension of μ₀ is .

Now the dimension of R.H.S of eq(iii) will be dimension of C × dimension of μ₀

dimension of

As the dimensions of electric resistance given by Ohm’s Law(V=iR) is

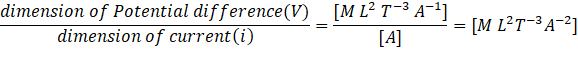
Therefore has the same dimensions as that of electrical resistance.

Also the value of is a constant because the R.H.S of eq(iii) is the

product of 2 universal constants.