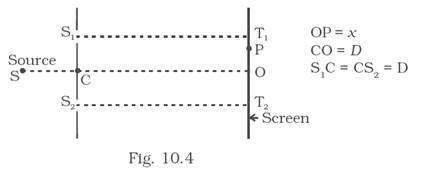
Consider a two slit interference arrangements (Fig. 10.4) such that the distance of the screen from the slits is half the distance between the slits. Obtain the value of D in terms of such that the first minima on the screen falls at a distance D from the centre O.

Question

Consider a two slit interference arrangements (Fig. 10.4) such that the distance of the screen from the slits is half the distance between the slits. Obtain the value of D in terms of such that the first minima on the screen falls at a distance D from the centre O.

Philoid · Accepted Answer

Given:

Young’s double slit experiment is shown in the figure.

The distance of the screen from the slit (D) is half the distance between the slits i.e.

S₁C = S₂C = D

Let the point of first minima or maxima be P; OP = x

If x = D, then we need to find the value of D in terms of λ for first minima.

Now, according to the young’s double slit experiments the path difference of the coherent waves is given as from the figure,

S₁P from the figure, in triangle S₁PT₁;

(1)

S₂P from the figure, in triangle S₂PT₂;

(2)

Path difference is given by subtracting the distance traversed by wave from source 2 from that traversed by wave originating from source 1,

(3)

According to the question the distance of the minima from the centre of the screen is equal to D. Therefore, putting the value of x in the in eq. (3) as x = D;

(4)

For minima (destructive interference) to occur at point P the path difference should be a multiple of (), that is,

First minima the value of m = 0

So, (5)

Now substituting the value of (4) in the (5)