Find the equivalent resistance of the circuits shown in figure between the points a and b. Each resistor has a resistance r.

(a)

Concepts/Formula used:
Resistors in Series:

Resistors in parallel:

The given circuit can be rewritten as

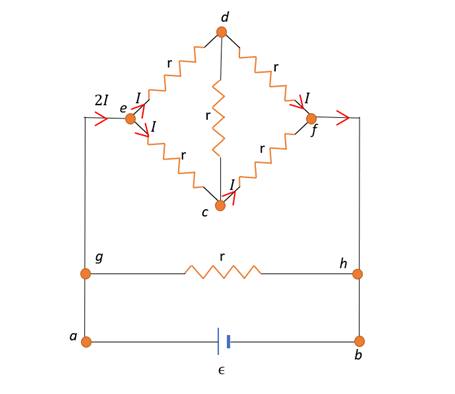
As we wish to find the resistance between points a and b, we have proceeded to add a voltage source of emf ϵ between the points a and b.

Let the net current passing through the upper branch be 2I. We can see that the upper branch is symmetric i.e. its upper and lower portion are the identical. Thus, the current should divide equally when branching out. So, current through ec and ed is I.

Now,

Hence,

As there is no potential difference across dc, there is no current passing through dc.

Hence, we can rewrite the circuit without the resistor across dc.

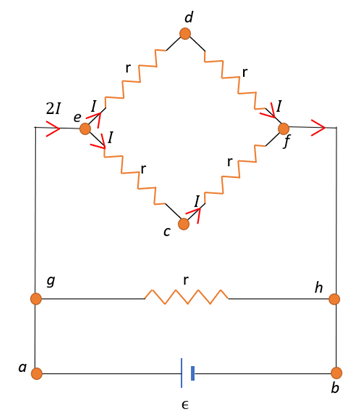
This circuit is equivalent to:

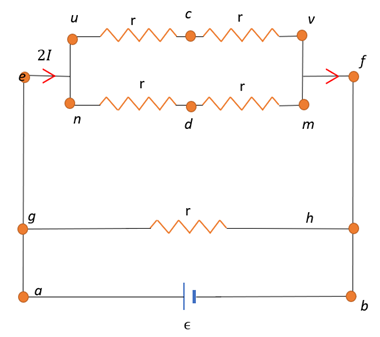
We can see that uc and cv are in series.

Also, nd and dm are is series.

Hence, we can rewrite the circuit as follows:

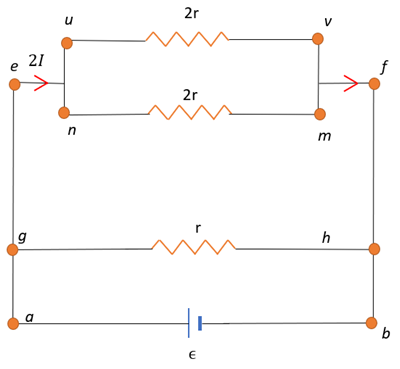
Clearly, uv and nm and gh are in parallel.

(b)

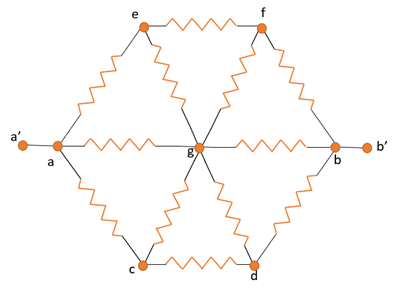
Note that because of symmetry,

Hence, we can rewrite the circuit as cg and gd are is series and eg and gf are in series.

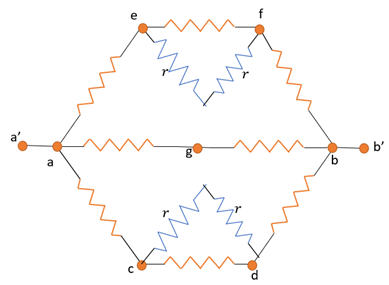
The blue resistors are in series. Net resistance =

Now, the blue resistors are in parallel.

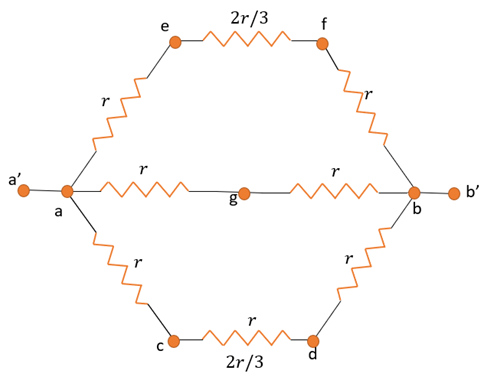
Now, the equivalent resistance of the upper branch and the lower branch is:

The middle branch:

Now, the circuit looks like this:

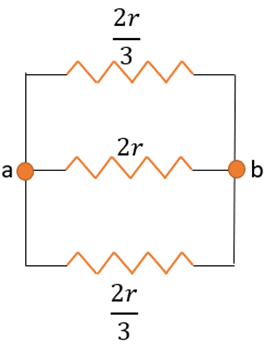
All the resistance are now in parallel:
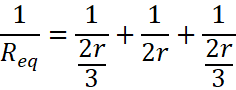