Draw the effective equivalent circuit of the circuit shown in Fig 7.1, at very high frequencies and find the effective impedance.

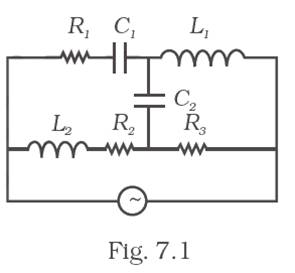
We know that the inductive reactance is given by:

where L is the inductance and f is the frequency. Hence at high frequency, current won’t pass through the inductor. (X_L acts like resistance). We can remove the branches with inductor.

Also, the capacitive reactance is:

where C is the capacitance ad f is the frequency. From the equation, we can see the inverse relation between capacitive reactance and frequency. Hence, increasing the frequency will increase decrease X_C. Thus, the current will easily pass through the capacitor. The capacitor will act like short circuit. Hence, we can replace the capacitors by pieces of wire.

The resistances are in series. Hence, the net resistance is:

Now, the impedance is given by: