Fig. 1.10 represents a crystal unit of cesium chloride, CsCl. The cesium atoms, represented by open circles are situated at the corners of a cube of side 0.40nm, whereas a Cl atom is situated at the centre of the cube. The Cs atoms are deficient in one electron while the Cl atom carries an excess electron.

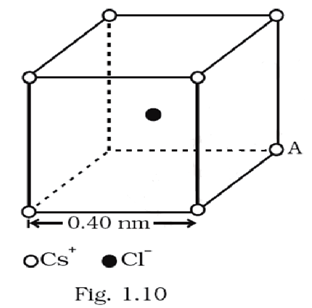
(i) What is the net electric field on the Cl atom due to eight Cs atoms?

(ii) Suppose that the Cs atom at the corner A is missing. What is the net force now on the Cl atom due to seven remaining Cs atoms?

i) The Diagonally opposite pair of charges (Cs⁺) will nullify each other’s electric field at the center of its diagonal.

So, the net electric field on the Cl atom due to eight Cs atoms will be zero.

Here,

Now, according to question..

Charge (q) and distance(r) are same so forces are same and in opposite direction . Therefore, they get cancelled out and net force becomes zero.

Now electric field E=. Here F=0 .Therefore, E is also zero.

Similarly, electric field in all directions are zero.

ii) We can deduce that electric field due to others 7 vertices (Cs⁺) and (Cs⁺) at A contributes at the center of its diagonal to get the electric field = 0

So we can write,

E_A + E’ =0

Where E_A = Electric field at center of cube due to charge at vertex a.

E’ = Electric field at center of cube due to charges at other 7 vertices.

∴E’ = - E_A

So the net force on Cl will be magnitude wise E_Aq(Cl^-)

where q = 1.6 and k = 9×10⁹ N.m².C⁻²

Diagonal of cube=, where a=length of side of a cube

Since Cl is placed at the centre 9×10⁹ N.m².C⁻²

r and

F= E_Aq(Cl^-)

It will be of attractive nature as the charges are of opposite nature and along the diagonal joining vertex A and the Centre.