Five charges, q each are placed at the corners of a regular pentagon of side ‘a’ (Fig. 1.12).

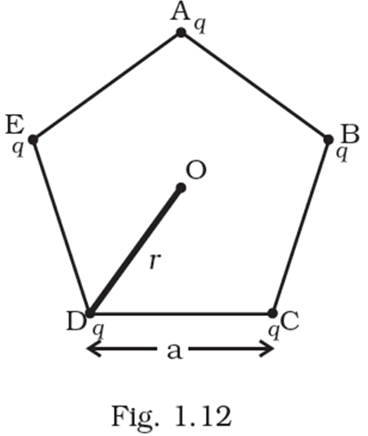
(a) (i) What will be the electric field at O, the center of the pentagon?

(ii) What will be the electric field at O if the charge from one of the corners (say A) is removed?

(iii) What will be the electric field at O if the charge q at A is replaced by –q?

(b) How would your answer to (a) be affected if pentagon is replaced by n-sided regular polygon with charge q at each of its corners?

Question

Five charges, q each are placed at the corners of a regular pentagon of side ‘a’ (Fig. 1.12).

(a) (i) What will be the electric field at O, the center of the pentagon?

(ii) What will be the electric field at O if the charge from one of the corners (say A) is removed?

(iii) What will be the electric field at O if the charge q at A is replaced by –q?

(b) How would your answer to (a) be affected if pentagon is replaced by n-sided regular polygon with charge q at each of its corners?

Philoid · Accepted Answer

a) i) As we can depict in the fig each charge is at equal distance from o and the magnitude of all the charge is same i.eq.

So by using the formula of electric field due to point charge we can get the magnitude of electric field at O by individual charges at vertex.

E = k

Where k = 9×10⁹ N �m² �C⁻²

The net electric field at O can be calculate with the help of vector and geometry.

As,

So the net Electric Field at point o in x direction

And the net Electric Field at point o in y direction

So net electric field at point O is Zero.

ii) As we solved in previous case, net electric field at point O is

E_A +E_B+E_C+E_D+E_E = 0 ........(1)

So If we Remove the charge from point A the net Electric field at point O will be due to

E_B+E_C+E_D+E_E and that is equal to (- E_A)Using eq;-1

So, the electric field at O if the charge from one of the corner A is removed will be (- E_A)= k j ∵ E_A = - k j

j is direction unit vector

iii) Now if the charge q at A is replaced by –q, It will be the improved part of ii) solution

As when there was no charge at A net electric field on point O was so adding –q charge will add an electric field of

So net Electric field will be

b) We can use complex nos. and geometry to answer it mathematicallyas we can see in 3 side regular polygon i.e equilateral Triangle the net E will be 0 at centroid,

And in even sided polygon like square or hexagon and so on the diagonally opposite charges will nullify there electric field resulting it to be zero.

And In odd sided polygon we can see in polygon the symmetry comes in role and eventually cancel out the effect of electric field of each other.

So no matter what and irrespective of no. of sides of polygon the net electric field due to N sided regular polygon at its geometrical center will be zero.