Two charges –q each are fixed separated by distance 2d. A third charge q of mass m placed at the mid-point is displaced slightly by x (x

Question

Two charges –q each are fixed separated by distance 2d. A third charge q of mass m placed at the mid-point is displaced slightly by x (x<<d) perpendicular to the line joining the two fixed charged as shown in Fig. 1.14. Show that q will perform simple harmonic oscillation of time period.

Philoid · Accepted Answer

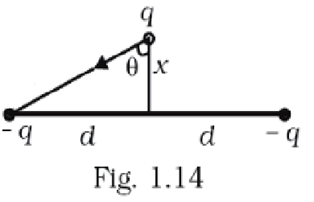
Now, drawing the free body diagram of q,

The sine components are equal and opposite in direction so they get cancelled. Adding the cosine components we get,

In the y direction we get,

----- eq. 1

Now

Substituting the values we get

Can also be written as

Since d²>>x²

We can Use binomial expansion,,where x<<1

F=

Since d²>>x², We can neglect

∴ As , F= ma

-ive sign justifies is acting towards the mean position

----eq.1

we find eq.1 is directly proportional to negative of distance from mean position.

So, the motion will be SHM ,

a=-x -------eq.2

Comparing eq.1 and eq.2

We get the value of

∴

As we know

∴

Hence proved.