In the circuit shown in Fig. 2.7, initially K1 is closed and K2 is open. What are the charges on each capacitor. Then K1 was opened and K2 was closed
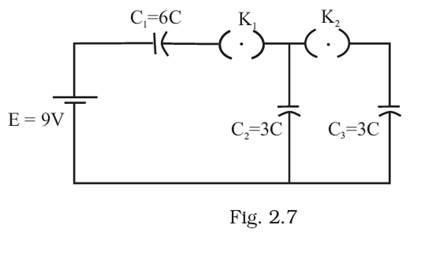
(order is important), What will be the charge on each capacitor now? [C = 1μF]
Given
Capacitance of capacitor C1 = 6C = 6μF
Capacitance of capacitor C2 = 3C = 3μF
Capacitance of capacitor C3 = 3C = 3μF
Voltage of battery E = 9V
When the switch K1 is closed and K2 is open, then the capacitors C1 and C �2 get charged and voltage V1 and V2 develops across the capacitors respectively. Applying Kirchhoff’s voltage law in the circuit, we get
![]()
![]()
We know that the charge stored in a capacitor and the voltage applied across it are related as:
![]()
![]()
Then for the capacitors C1 and C2,
![]()
![]()
Then from equations (1) and (2) we have
![]()
![]() and
and
![]()
Now the charges on the capacitors
![]() and
and
![]()
After this, when K1 was opened and K2 is closed the capacitor C2 becomes parallel to C3.
By conservation of charge we have
![]()
Where Q2’ is the new charge on capacitor C2 and Q3 is the charge flown from C2 to C3. Since they are connected in parallel, they have a common potential difference across them. Let the common potential be V’, then
![]()
![]()
Therefore, the charges on the capacitors are:
![]() and
and
![]()
Therefore, the final charges on all the capacitors are:
![]() ,
,
![]() and
and
![]()