If ABC and DEF are similar triangles such that = 47° and = 83°, then =

Question

Philoid · Accepted Answer

Given ABC and DEF are two similar triangles, ∠A = 47° and ∠E = 83°

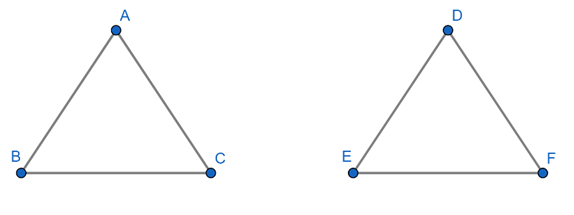
We know that SAS similarity criterion states that if one angle of a triangle is equal to one angle of the other triangle and the sides including these angles are proportional, then the two triangles are similar.

In ΔABC and ΔDEF,

if and ∠A = ∠D, then ΔABC ~ ΔDEF

So, ∠A = ∠D

⇒ ∠D = 47° … (1)

Similarly, ∠B = ∠E

⇒ ∠B = 83° … (2)

We know that the sum of all angles of a triangle is equal to 180°.

⇒ ∠A + ∠B + ∠C = 180°

⇒ 47° + 83° + ∠C = 180°

⇒ 130° + ∠C = 180°

⇒ ∠C = 180° - 130° = 50°

∴ ∠C = 50°