Solve the previous problem if the friction coefficient between the 2.0 kg block and the plane below it is 0.5 and the plane below the 4.0 kg block is frictionless.
Given
The radius of the pulley is given as 10 cm, the inertia 0.5 
and the mass of the blocks are given as 2 kg and 4 kg and an additional friction of 0.5 under the block of 2 kg mass.
Formula Used
The formula used to find the acceleration of the mass pulled/pushed is determined by the second law of Newton when the Force/Tension applied is equivalent to the product of mass and acceleration
where
F is the force of the mass in terms of tension, 
is the coefficient of friction and N is the reactionary force of the block.
Explanation
The mass and tension relationship of the 4 kg block is given as
The tension and the mass of the 4 kg block is denoted as 
and 
respectively.
The mass and tension relationship of the 2 kg block is given as
The tension and the mass of the 2 kg block is denoted as 
and 
respectively.
The difference in their tension is given in terms of moment of Inertia
Adding all the three equations we get the value of the acceleration of the blocks made the 4 kg block is
Putting the values of the masses, radius, friction and gravity as 
, we get the value of acceleration as
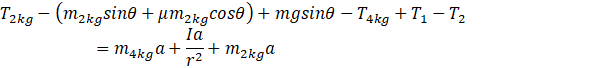
