A uniform rod of length L rests against a smooth roller as shown in figure (10-E9). Find the friction coefficient between the ground and the lower end if the minimum angle that the rod can make with the horizontal is θ.

The friction applied if the angle is minimum is

Given

The length of the rod is given as L, kept at an angle of θ at a height of h

Formula Used

The torque applied by the mass and radius of the rod is the product of the force and the radius of rotation of the rod falling

where

is the torque of the rod, F is the force in terms of tension, r is the length of the weight suspended at certain distance, is the angular turning distance of the rod is rotated, is the friction applied.

Explanation

According to the diagram given below let us find the value of the reactionary forces at the top of the rod which is

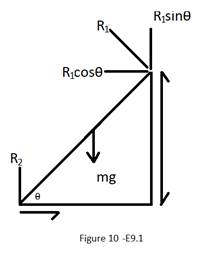
The vertical and the horizontal component of the reactionary forces of the rod is

and …… (1)

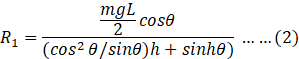
Now placing the value of the coefficient of friction in the above equation of , we get

Replacing the with the above equation (2) and placing