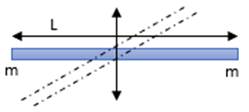
Two small balls A and B, each of mass m, are joined rigidly to the ends of a light rod of length L (figure 10-E10). The system translates on a frictionless horizontal surface with a velocity in a direction perpendicular to the rod. A particle P of mass m kept at rest on the surface sticks to the ball A as the ball collides with it. Find

(a) the linear speeds of the balls A and B after the collision, (b) the velocity of the center of mass C of the system A + B + P and (c) the angular speed of the system about C after the collision.

[Hint: The light rod will exert a force on the ball B only along its length.]

Given:

Mass of each ball = m

Length of the rod= L

Velocity of the system=

(a) collision pf the particle P with the ball A will not affect the velocity of the ball because the force exerted by the rod is along the length

Velocity of B =

Using the law of conservation of linear momentum, we get,

Velocity of ball A=

(b) consider the system of three bodies as one single system

Not external force is acting on the system

Hence,

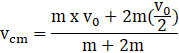
(c) velocity of particle P and the ball A with respect to the center of the mass

Velocity of ball B with respect to center of the mass=

Distance of the particle P and ball A from the center of mass =

Distance of ball B from the center of mass=

For angular speed