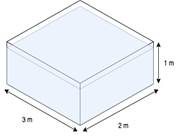
Water is filled in a rectangular tank of size 3 m × 2 m × 1 m. (a) Find the total force exerted by the water on the bottom surface of the tank. (b) Consider a vertical side of area 2 m × 1 m. Take a horizontal strip of width δx meter in this side, situated at a depth of x meter from the surface of water. Find the force by the water on this strip (c) Find the torque of the force calculated in part (b) about the bottom edge of this side. (d) Find the total force by the water on this side. (e) Find the total torque by the water on the side about the bottom edge. Neglect the atmospheric pressure and take g = 10 m s^-2.

(a)Given: Length, ; Breadth, ; Height, .

The pressure at the bottom of tank, —————(1)

Where is the density of water and g is the acceleration due to gravity,

—————(2)

Area of the bottom of the tank,

Therefore, Force, (ANS) —————(3)

(b) Here we first find the pressure at a depth of “x m” from the top.

We know, pressure,

The area of the strip is,

Therefore, the force on the strip is, ————(4)

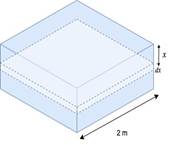
(c) To find the torque, we need to find the perpendicular distance(d) of the strip from the bottom edge.

We know, Torque, —————(5)

(d) To find the total force, we integrate the force in (b) for the entire height.

—————(6)

(e) The torque on the side can be found by integrating (5).

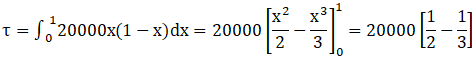
(ANS)