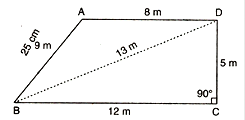
A park, in the shape of a quadrilateral ABCD, has C=90°, AB = 9 m, BC = 12 m, CD = 5 m and AD = 8 m. How much area does it occupy?
Let consider a quadrilateral ABCD
In ∆BCD;
BD = 
= 13 m
BC = a = 12 m, CD = b = 5 m, BD = c = 13 m
Let a, b and c are the sides of triangle and s is
the semi-perimeter, then its area is given by:
A = 
where 
[Heron’s Formula]
= 
= 15
A1 = 
A1 = 
= 
m2
In ∆ABD;
AB = a = 9 m, AD = b = 8 m, BD = c = 13 m
Let a, b and c are the sides of triangle and s is
the semi-perimeter, then its area is given by:
A = 
where 
= 
= 15
A2 = 
A2 = 
= 
m2
Therefore area of quadrilateral ABCD = A1 + A2 = 30+35.50 = 65.50 m2
4