In a ![]() , D and E are points on the sides AB and AC respectively such that
, D and E are points on the sides AB and AC respectively such that ![]()
(i) If AD = 6 cm, DB = 9 cm and AE = 8 cm, find AC.
(ii) If ![]() and AC = 15 cm, find AE.
and AC = 15 cm, find AE.
(iii) If ![]() and AC = 18 cm, find AE.
and AC = 18 cm, find AE.
(iv) If AD = 4, AE = 8, DB = x – 4, and EC = 3x – 19, find x.
(v) If AD = 8 cm, AB = 12 cm and AE = 12 cm, find CE.
(vi) If AD = 4 cm, DB = 4.5 cm and AE = 8 cm, find AC.
(vii) If AD = 2 cm, AB = 6 cm and AC = 9 cm, find AE.
(viii) If ![]() and EC = 2.5 cm, find AE.
and EC = 2.5 cm, find AE.
(ix) If AD = x, DB = x – 2, AE = x + 2 and EC = x – 1, find the value of x.
(x) If AD = 8x - 7, DB = 5x – 3, AE = 4x - 3 and EC = (3x – 1), find the value of x.
(xi) If AD = 4x – 3, AE = 8x – 7, BD = 3x – 1 and CE = 5x - 3, find the volume x.
(xii) If AD = 2.5 cm, BD = 3.0 cm and AE = 3.75 cm, find the length of AC.
(i)
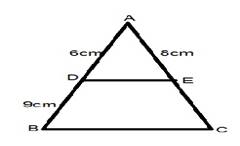
we have
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
6/9=8/EC
2/3=8/EC
EC=3x8/2
EC=3x4
EC=12 cm
(ii)

we have
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
Adding 1 both side
AD/DB +1=AE/EC +1
3/4 +1=AE+BC/BC
3+4/4=AC/EC [AE+EC=AC]
7/4= 15/EC
EC=15x4/7
EC=60/7
Now AE+EC=AC
AE+60/7=15
AE=15-60/7
AE=105-60/7
AE=45/7
AE=6.43 cm
(iii)
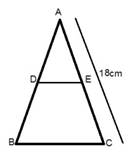
we have
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
Adding 1 both side
AD/DB +1=AE/EC +1
![]() +1=
+1=![]() +1
+1
![]() =
= ![]()
![]() = AC/AE [AE+EC=AC]
= AC/AE [AE+EC=AC]
5/2=18/AE
AE= ![]()
AE=36/5
AE=7.2 cm
(iv)
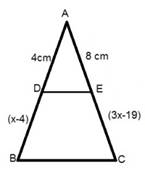
we have
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
![]() =
=![]()
4(3x-19)=8(x-4)
12x-76=8x-32
12x-8x=76-32
4x=44
x=44/4
x=11 cm
(v)

AD=8cm,AB=12cm
since BD=AB-AC
BD=12-8
BD=4 cm
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
8/4=12/EC
EC=![]()
EC =6 cm
(vi)

we have
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
4/4.5=8/EC
EC=![]()
EC=9cm
Now AE+EC=AC
AC=8+9
AC=17 cm
(vii)
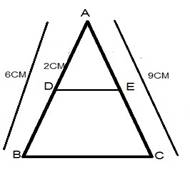
AD=2cm, AB=6cm
Since BD=AB-AC
BD=6-2
BD=4 cm
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
Taking reciprocal on both side
DB/AD=EC/AE
4/2=EC/AE
Adding 1 both side
AD/DB +1=AE/EC +1
![]() +1=
+1=![]() +1
+1
![]() =
= ![]()
![]() = AC/AE [AE+EC=AC]
= AC/AE [AE+EC=AC]
3=9/AE
AE= ![]()
AE=3 cm
(viii) we have
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
4/5=AE/2.5
AE=4x2.5/5
AE=10/5
AE=2 cm
(ix) we have
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
![]() =
=![]()
x(x-1)=(x+2)(x-2)
x2-x=x2-22
-x=-4
x=4 cm
(x) we have
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
![]() =
=![]()
(8x-7)(3x-1)=(4x-3)(5x-3)
8x(3x-1)-7(3x-1)=4x(5x-3)-3(5x-3)
24x2-8x-21x+7=20x2-12x-15x+9
24x2-20x2-29x+27x+7-9=0
4x2-2x-2=0
2[2x2-x-1]=0
2x2-x-1=0
2x2-2x-x-1=0
2x(x-1)+1(x-1)=0
(x-1)(2x+1)=0
x-1=0
x=1
or 2x+1=0
or x=-1/2
-1/2 is not possible.
So x=1
(xi) we have
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
![]() =
=![]()
(8x-7)(3x-1)=(4x-3)(5x-3)
24x2-8x-21x+7=20x2-12x-15x+9
24x2-20x2-29x+27x+7-9=0
4x2-2x-2=0
2[2x2-x-1]=0
2x2-x-1=0
2x2-2x-x-1=0
2x(x-1)+1(x-1)=0
(x-1)(2x+1)=0
x-1=0
x=1
or 2x+1=0
or x=-1/2
-1/2 is not possible.
So x=1
(xii) we have
DE![]() BC
BC
Therefore by basic proportionally theorem
AD/DB=AE/EC
2.5/3=3.75/EC
EC=3.75x3/2.5
EC=375x3/250
EC=15x3/10
EC=9/2
EC=4.5 cm
Now AC=AE+EC
AC=3.75+4.5
AC=8.25 cm