In Fig. 4.145 (a) is right angled at C and . Prove that and hence find the lengths of AE and DE.

Question

Philoid · Accepted Answer

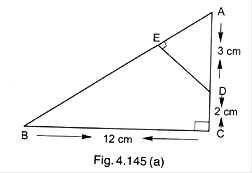
In ΔABC, by Pythagoras theorem

AB² = AC² + BC²

Or, AB² = 5² + 12²

Or, AB² = 25 + 144

Or, AB² = = 169

Or AB = 13 (Square root both side)

In Δ AED and Δ ACB

Then, Δ AED ~ Δ ACB(Corresponding parts of similar triangle are proportional)

So, AE/AC = DE/ CB =AD/ AB

Or, AE/5 = DE/12 = 3/13

Or, AE/5 = 3/13 and DE/12 = 3/13

Or, AE = 15/13cm and DE = 36/13cm