The shadow of a tower, when the angle of elevation of the sun is 45°, is found to be 10 m longer than when it was 60°. Find the height of the tower.

Let the height of the tower = h (m)

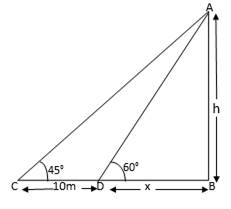
Let the point of 60° elevation is (m) away from the foot of the tower.

In ∆ABC,

tan 45° =

1 =

1 =

h = 10+ ----(1)

In ∆ABD,

tan 60° =

√3 =

h = √3

= ---------(2)

From eqn. (2) in eqn. (1)

h =

h - = 10

= 10

= 10√3

⇒

h= ⇒ h=

⇒ 15+5√3 ⇒ 23.66 m.

Therefore height of the tower is 23.66 m.