A carpenter makes stools for electricians with a square top of side 0.5 m and at a height of 1.5 m above the ground. Also, each leg is inclined at an angle of 60° to the ground. Find the length of each leg and also the lengths of two steps to be put at equal distances.

Question

Philoid · Accepted Answer

In the fig let the height of the stool from the ground is 1.5m i.e. AL = BM = 1.5m

In ∆ACL

tan 60° =

√3 =

CL = ⇒

⇒ = 0.866m

Again in ∆ACL

Sin 60° =

=

AC = = √3m ⇒ 1.732m

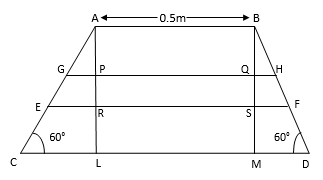
Lengths of steps are GH and EF and steps are put at equal distance such that AP =PR = RL

Now consider ∆AGP and ∆ACL

=

GP = 0.2887m

Length of step = GH = GP + PQ + QH = GP + AB + GP (Since QH = GP)

GH = 2GP + AB = 2 × 0.2887 + 0.5 = 1.0774m

Similarly we can say that,

ER = ⇒ = 0.577m

Length of the step is EF = ER + RS + SF

⇒ ER + AB + ER = 2ER + AB

EF = 2× 0.5773 + 0.5 ⇒ 1.6546m