A straight highway leads to the foot of a tower of height 50 m. From the top of the tower, the angles of depression of two cars standing on the highway are 30° and 60° respectively. What is the distance between the two cars and how far is each car from the tower?

In the fig AB is the tower on the highway.

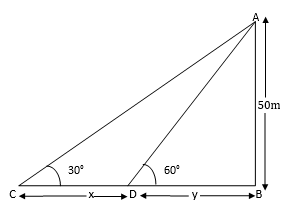
In ∆ABC

tan 30° =

=

x + y = 50√3 ………………..(1)

In ∆ABD

tan 60° =

√3 =

y =

On multiplying and dividing by √3, we get

y = ⇒ ………………(2)

Therefore the distance between

the first car and tower is 28.87m

On substituting value of y

from eqn (2) in eqn (1)

x + = 50√3

x = 50√3 -

⇒

⇒ = = 57.73m

The distance between two cars is 57.73m

The distance between second car and tower is (x + y) = 57.73 + 28.87 = 86.60m