Find the ratio in which the line segment joining (-2, -3) and (5, 6) is divided by (i) x-axis (ii) y-axis. Also, find the coordinates of the point of division in each case.
(i) x-axis
(i) x-axis
Let our points be A(-2, -3) and B(5, 6).
Let point C(x, 0) divide the line formed by joining by the points A and B in ratio of m:n.
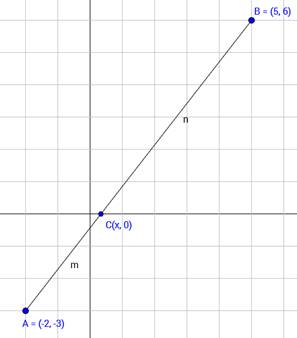
By section formula,
x = ![]() , y =
, y = ![]()
For point C(x, 0)
x = ![]() , 0 =
, 0 = ![]()
Solving for y coordinate,
0 = ![]()
∴ 6m -3n = 0
∴ 2m = n
∴ ![]() =
= ![]()
∴ m : n = 1 : 2
Now solving for x coordinate, with m = 1 and n = 2,
x = ![]()
∴ x= ![]()
∴ x = ![]()
Hence, the coordinates of required point is C(![]() , 0)
, 0)
(ii) y-axis.
Let our points be A(-2, -3) and B(5, 6).
Let point C(0, y) divide the line formed by joining by the points A and B in ratio of m:n.

By section formula,
x = ![]() , y =
, y = ![]()
For point C(0, y)
0 = ![]() , y =
, y = ![]()
Solving for x coordinate,
0 = ![]()
∴ 5m – 2n = 0
∴ ![]() =
= ![]()
∴ m : n = 2 : 5
Now solving for y coordinate, with m = 2 and n = 5,
y = ![]()
y = ![]()
∴ y = ![]()
Hence, the coordinates of required point is C(![]() ,
, ![]() )
)