Look at the following matchstick pattern of squares (see figure). The squares are not separate. Two neighbouring squares have a common matchstick. Observe the pattern and find the rule that gives the number of matchsticks in terms of the number of squares.

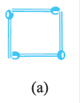
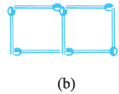
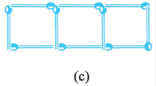
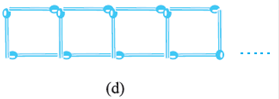
From the above given figures it can be observed that,

The number of matchsticks in the above given pattern are 4, 7, 10 and 13 respectively

i.e., The matchstick are 1 more than the thrice of the number of squares in the pattern

Hence,

The pattern for above given figures will be:

3n + 1

Where n is the number of squares