In the given figure, BOC is a diameter of a circle and AB = AC. Then, ∠ABC = ?

Question

In the given figure, BOC is a diameter of a circle and AB = AC. Then, ∠ ABC = ?

Philoid · Accepted Answer

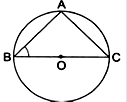
Given: BOC is the diameter of the circle

AB = AC

Here, BAC forms a semicircle.

We know that angle in a semicircle is always 90

BAC = 90

Here ABC = ACB (since angles opposite equal sides are equal in a triangle)

We know that sum of all the angles in the triangle is 180

That is

ABC + ACB + BAC = 180

⇒ 2 × ABC + BAC = 180

⇒ 2 × ABC + 90 = 180

⇒ 2 × ABC = 180 – 90

⇒ 2 ×ABC = 90

⇒ ABC = 45