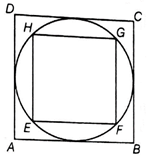
In figure, a square is inscribed in a circle of diameter d and another square is circumscribing the circle. Is the area of the outer square four times the area of the inner square? Give reason for your answer.
False
Diameter of the circle = d
Therefore,
Diagonal of inner square EFGH = Side of the outer square ABCD = Diameter of circle = d
Let side of inner square EFGH be a
Now in right angled triangle EFG,
(EG)2 = (EF)2 + (FG)2
By Pythagoras theorem)
⇒ d2 = a2 +a2
⇒ d2 = 2a2
⇒ a2 = d2/2
∴ Area of inner circle = a2 = d2/2
Also, Area of outer square = d2
∴ the area of the outer circle is only two times the area of the inner circle.
Thus, area of outer square is not equal to four times the area of the inner square.
3