In figure, a square of diagonal 8 cm is inscribed in a circle. Find the area of the shaded region.
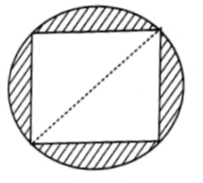
Let a be the side of square.
∴ Diameter of a circle = Diagonal of the square = 8 cm
Now in right angled triangle ABC,
(AC)2 = (AB)2 + (BC)2
(By Pythagoras theorem)
∴ (8)2= a2 +a2
⇒ 64= 2a2
⇒ a2= 32
Hence area of square = a2= 32 cm2
∴ Radius of the circle = Diameter/2 = 4 cm
∴ Area of the circle = πr2 = π(4)2 = 16 cm2
So, the area of the shaded region = Area of circle – Area of square
∴ the area of the shaded region = 16π – 32
= 16 × (22/7) – 32
= 128/7
= 18.286 cm2
2