In figure, AB is a diameter of the circle, AC = 6 cm and BC = 8 cm. Find the area of the shaded region. (use π = 3.14)
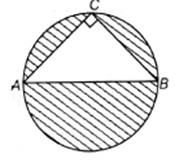
Given, AC = 6cm and BC = 8 cm
A triangle in a semi-circle with hypotenuse as diameter is right angled triangle.
∴ In right angled triangle ACB,
(AB)2 = (AC)2 + (CB)2
(By Pythagoras theorem)
(AB)2 = (6)2 + (8)2
⇒(AB)2 = 36 + 64
⇒(AB)2 = 100 ⇒(AB)= 10
∴ Diameter of the circle = 10 cm
Thus, Radius of the circle = 5 cm
Area of circle = πr2
= π(5)2
= 25π cm2
= 25 × 3.14 cm2
= 78.5 cm2
Also, Area of the right angled triangle = (1/2) × Base × Height
= (1/2) × AC × CB
= (1/2) × 6 × 8 = 24 cm2
Now, Area of the shaded region = Area of the circle – Area of the triangle
= (78.5-24)cm2
= 54.5cm2
7