In figure arcs are drawn by taking vertices A, B and C of an equilateral triangle of side 10 cm, to intersect the sides BC, CA and AB at their respective mid-points D, E and F. Find the area of the shaded region. (use π = 3.14)
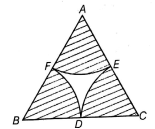
Since D, E, F bisects BC, CA, AB respectively.
∴ AE = EC = CD = DB = BF = FA = 5 cm
Now area of the shaded region = (Area of the three sectors)
Since the triangle is an equilateral triangle, therefore each angle is of 60°
∴ Angle subtended at the center of each sector = 60°
Angle subtended at the center (in radians) = θ = 62π/180 = π/3
Radius of each sector = 5 cm
∴ Area of a sector of a circle ![]()
![]()
![]()
∴ Area of three sectors of a circle ![]()
= 78.5/2 cm2
= 39.25 cm2
∴ Area of shaded region = 39.25 cm2
12