All the vertices of a rhombus lie on a circle. Find the area of the rhombus, if area of the circle is 1256 cm². (use π = 3.14)

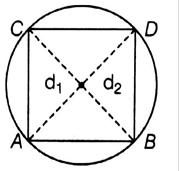
Are of the circle = 1256 cm²

Let r be the radius of the circle.

∴ Area = πr²⇒ 1256 = 3.14 × r²⇒ r² = 1256/3.14 = 400

⇒ r = 20 cm

∴ Diameter of the circle = d = 2×20 = 40 cm

Since all the vertices of a rhombus lie on a circle, therefore the diagonals of the rhombus pass through the center of the circle and thus diagonals of the rhombus are equal to the diameter of the circle.

Let d₁ and d₂ be the diagonal of the rhombus.

Since, diagonals of a rhombus are equal, therefore d₁ = d₂ = d = 40 cm

Now, Area of the rhombus = (1/2) × d₁ × d₂

= (1/2) × 40 × 40 = 800 cm²

Hence, the required area of rhombus is 800 cm².