Find the area of the shaded region given in figure.

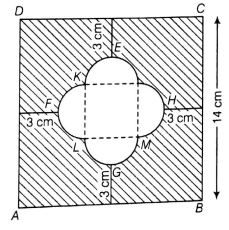
ABCD is a square of side 14 cm.

Inside the square ABCD, there are four congruent semi-circles, and thus forming the square JKLM inside the outer square.

∴ Area of the shaded region = Area of the square ABCD – (Area of the square JKLM + Area of the four congruent semi-circles)

Area of the outer square ABCD = (Side)² = (14)² = 196cm²

Now, there are four congruent semi-circles and JKLM forms a square.

∴ EG = FM = (14-3-3) cm = 8 cm

So, the side of square should be 4 cm and radius of semi-circle of both ends are 2 cm each.

Area of each semi-circle = (1/2)r²θ

= (1/2) × (22/7) × 4

= 2π = 44/7 cm²

Area of the square JKLM = (Side)² = 16 cm²

∴ Area of the shaded region = 196 – (16 + 4(2π)) = (180 – 8π) cm²