There are two scales of measuring temperature, namely, Fahrenheit (F) and Celsius (C).

The relation between the two scales is given by

(i) Draw the graph of the given linear equation taking C along x-axis and F along y-axis.

Fill in the blanks given below:

(ii) 0°C = (…)°F (iii) 95°F = (…)°C

(iv) 0°F = (…)°C

(v) Find the temperature which is numerically the same in both (F) and (C).

(i) Given equation,

Let, then,

⇒ F = 0 + 32

⇒ F = 32°

Let, then,

⇒ F = 18 + 32

⇒ F = 50°

Let, then,

⇒ F = 36 + 32

⇒ F = 68°

On plotting, (0, 32), (10, 50) and (20, 68) we get the following graph,

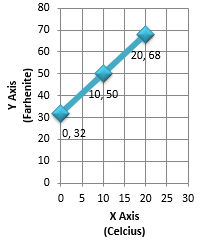
The blue line indicates the required graph of

(ii) When,, then,

⇒ F = 0 + 32

⇒ F = 32°

(iii) When , then

⇒ C = 35°

(iv) When F = 0°, then

⇒ C = 17.7°

(v) Put C = F, then

⇒ F = – 40° = C

Therefore,– 40°F = – 40°C