In figure, two-line segments AC and BD intersect each other at the point P such that PA = 6 cm, PB = 3 cm, PC = 2.5 cm, PD = 5 cm, ∠APB = 50° and ∠CDP = 30°. Then, ∠PBA is equal to

Question

In figure, two-line segments AC and BD intersect each other at the point P such that PA = 6 cm, PB = 3 cm, PC = 2.5 cm, PD = 5 cm, ∠ APB = 50° and ∠ CDP = 30°. Then, ∠ PBA is equal to

Philoid · Accepted Answer

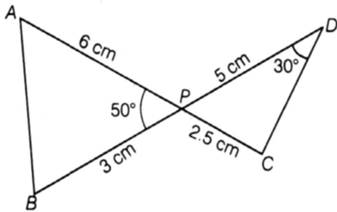
In ∆APB and ∆CPD,

∠APB = ∠CPD = 50° (vertically opposite angles)

AP/PD = 6/5 …(i)

Also, BP/CP = 3/2.5

Or BP/CP = 6/5 …(ii)

From equations (i) and (ii)

AP/PD = BP/CP

∴ ∆APB ∼ ∆DPC [by SAS similarity criterion]

∴ ∠A = ∠D = 30° [corresponding angles of similar triangles]

In ∆APB,

∠A + ∠B + ∠APB = 180°

[Sum of angles of a triangle = 180°]

⇒ 30° + ∠B + 50° = 180°

∴ ∠B = 180° - (50° + 30°)

∠B = 180 – 80° = 100°

∠PBA = 100°