Two solid cones A and B are placed in a cylindrical tube as shown in the figure. The ratio of their capacities is 2 : 1. Find the heights and capacities of cones. Also, find the volume of the remaining portion of the cylinder.
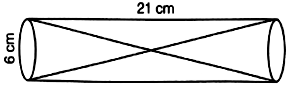
The diagram is:

Diameter of Cylinder = 6 cm
Radius of cylinder = r = 3 cm
[As radius = diameter/2]
As both cones have equal radius
Radius of cone A = radius of cone B = r = 3 cm
Let the height of cone A be h1 and Cone B be h2
Given,
Ratio of volume of cones is 2 : 1
i.e.
![]()
As volume of cone = ![]()
where r = base radius and h = height
⇒ ![]()
⇒ h1 = 2h2
Now,
Total height of cylinder is 21 cm
h1 + h2 = 21
2h2 + h2 = 21
3h2 = 21
h2 = 7 cm
h1 = 2h2 = 2(7) = 14 cm
![]()
![]()
![]()
![]()
We know,
Volume of cylinder = πr2h ,
where r = radius and h = height
Volume of given cylinder = π(3)2(21)
![]()
Volume of remaining solid = (Volume of cylinder) – (volume of cone A) – (volume of cone B)
= 594 - 132 - 66 = 396 cm3