If cosθ = 7/25, find all the values of all trigonometric ratios of θ.
We have, cosθ = (7k)/(25k) = base/hypotenuse (For some value of k)
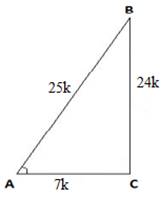
By Pythagoras theorem, (hypotenuse)2 = (perpendicular)2 + (base)2
∴AB2 = BC2 + AC2
= (25k)2 = BC2 + (7k)2 (for some value of k)
= 625k2 = BC2 + 49k2
= BC2 = 576k2
= BC2 = (24k)2
→ BC = 24k
Hence, the trigonometric ratios of the given θ are:
sinθ = BC/AB = (24k)/(25k) = 24/25
cosθ = 7/25
tanθ = BC/AC = sinθ /cosθ = 24/7
cotθ = AC/BC = 1/tanθ = 7/24
cosecθ = AB/BC = 1/sinθ = 25/24
secθ = AB/AC = 1/cosθ = 25/7
2